NO.PZ2020011303000242
问题如下:
Calculate the forward bucket 01s for a two-year bond with a coupon of 8% and a face value of USD 10,000 when the there are two buckets: 0–1 year and 1–2 year. Assume that the term structure is flat at 4% (semi-annually compounded).
选项:
解释:
The value of the bond is
400/1.02+400/1.022 +400/1.023 +10,400/1.024 = 10,761.5457
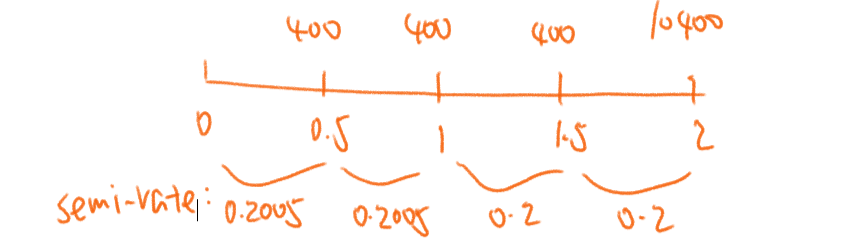
When the forward rates in the first bucket increase by one basis point, the value of the bond becomes
400/1.02005+400/1.020052 +400/(1.020052×1.02)+10,400/(1.020052×1.022)= 10,760.5100
This is a decrease of 1.0358. When the forward rates in the second bucket increase by one basis point, the value of the bond becomes
400/1.02+400/1.022+400/(1.022×1.02005)
+10,400/(1.022×1.020052) = 10,760.5854
This is a decrease of 0.9604. The forward bucket 01s are therefore 1.0358 and 0.9604.
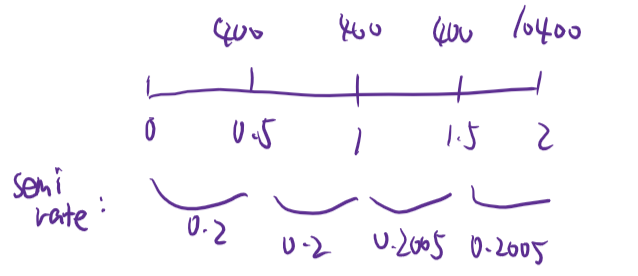
When the forward rates in the first bucket increase by one basis point, the value of the bond becomes
400/1.02005+400/1.020052 +400/(1.020052×1.02)+10,400/(1.020052×1.022)= 10,760.5100
第三期和第四期的分母为什么是这样的?还有后面这一步也不太明白。
This is a decrease of 1.0358. When the forward rates in the second bucket increase by one basis point, the value of the bond becomes
400/1.02+400/1.022+400/(1.022×1.02005)
+10,400/(1.022×1.020052) = 10,760.585