C方法1和讲义上的方法2

问题如下：

A European equity composite contains three portfolios. For convenience, the cash flow weighting factors are presented below.

A. Calculate the returns of Portfolio A, Portfolio B, and Portfolio C for the month of August using the Modified Dietz formula.

B. Calculate the August composite return by asset-weighting the individual portfolio returns using beginning-of-period values.

C. Calculate the August composite return by asset-weighting the individual portfolio returns using a method that reflects both beginning-of-period values and external cash flows.

选项：

解释：

A.

Portfolio returns:

${l}r_A=\frac{85.3-74.9-7.5}{74.9+(7.5\times0.613)}=\frac{2.9}{79.5}=0.0365=3.65\%\\$

$r_B=\frac{109.8-127.6-(-15)-(-5)}{127.6+(-15\times0.742)+(-5\times0.387)}=\frac{2.2}{114.535}=0.0192=1.92\%$

$r_C=\frac{128.4-110.4-15}{110.4+(15\times0.387)}=\frac3{116.205}=0.0258=2.58\%$

B.

To calculate the composite return based on beginning assets, first determine the percent of beginning composite assets represented by each portfolio; then determine the weighted-average return for the month:

Beginning composite assets = 74.9 + 127.6 + 110.4 = 312.9

Portfolio A = 74.9/312.9 = 0.239 = 23.9%

Portfolio B = 127.6/312.9 = 0.408 = 40.8%

Portfolio C = 110.4/312.9 = 0.353 = 35.3%

                              ${l}r_{Comp}=(0.0365\times0.239)+(0.0192\times0.408)+(0.0258\times0.353)\\=0.0257=2.57\%$

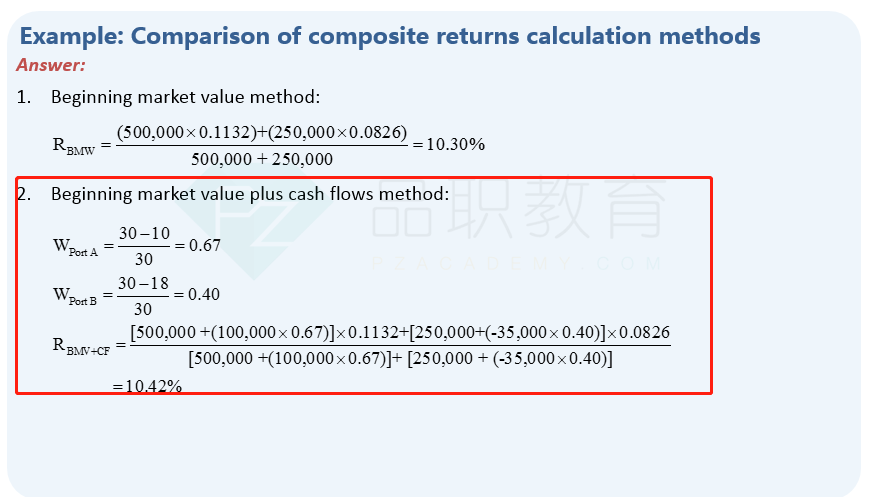
C.

To calculate the composite return based on beginning assets plus cash flows, first use the denominator of the Modified Dietz formula to determine the percentage of total beginning assets plus weighted cash flows represented by each portfolio, and then calculate the weighted-average return:

Beginning composite assets + Weighted cash flows = [74.9 + (7.5 × 0.613)] + [127.6 + (–15 × 0.742) + (–5×0.387)] + [110.4 + (15 × 0.387)] = 79.5 + 114.535 + 116.205 = 310.24

Portfolio A = 79.5/310.24 = 0.256 = 25.6%

Portfolio B = 114.535/310.24 = 0.369 = 36.9%

Portfolio C = 116.205/310.24 = 0.375 = 37.5%

${l}r_{Comp}=(0.0365\times0.256)+(0.0192\times0.369)+(0.0258\times0.375)\\=0.0261=2.61\%$

A mathematically equivalent method consists simply in summing beginning assets and intra-period external cash flows, treating the entire composite as though it were a single portfolio and then computing the return directly with the Modified Dietz formula.

${l}r_{Comp}=\frac{323.5-312.9-(-15+7.5+10)}{312.9+\lbrack(-15)\times0.742+7.5\times0.613+10\times0.387]}\\=0.0261=2.61\%$