开发者:上海品职教育科技有限公司 隐私政策详情

应用版本:4.2.11(IOS)｜3.2.5(安卓)APP下载

返回

海胆君 · 2021年03月20日

这道题能不能直接用St属于logN函数？

  1. FRM I
  2. Financial Markets And Products

NO.PZ2020021205000061

问题如下：

A stock has an expected return of 15% and a volatility of 20%. The current price of the stock is USD 50, estimate 99% confidence for the price in six months.

选项：

解释：

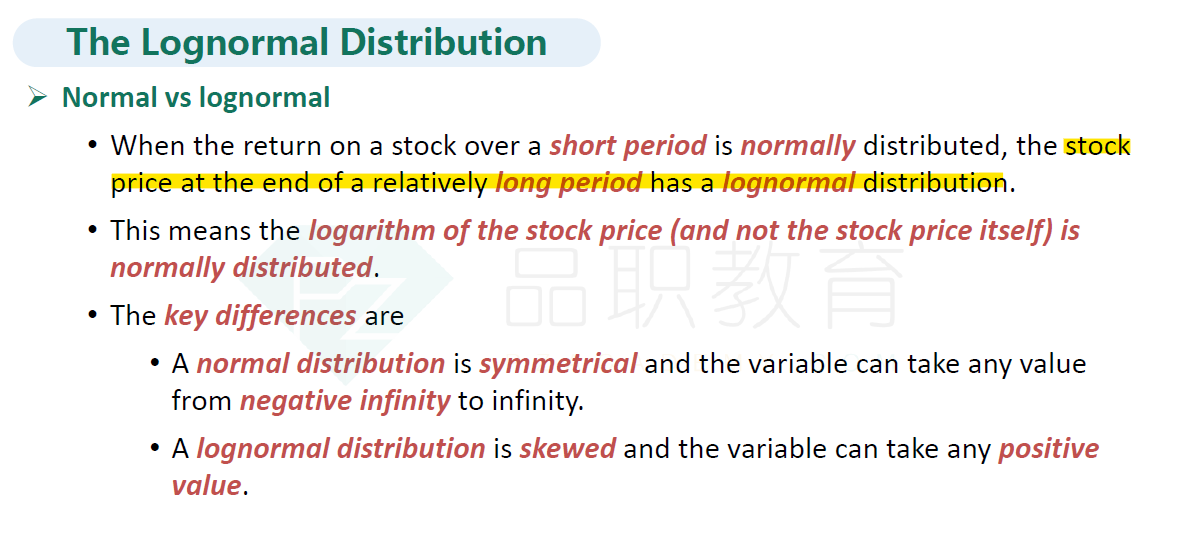
Here, the time period is longer, and we should work

with lognormal distributions. From Equations (15.2) and

(15.3), the logarithm of the stock price has mean:

ln(50) + (0.15 - 0.220.2^2/2) x 0.5 = 3.9770

and standard deviation:

0.2*0.5\sqrt{0.5} = 0.1414

We are 99% certain that:

3.9770 - N−1N^{-1}(0.995) x 0.1414 < ln(Sr) < 3.9770

+ N−1N^{-1} (0.995) X 0.1414

or

3.6127 < ln(Sr) < 4.3413

so that:

e3.6127e^{3.6127} < Sr < e4.3413e^{4.3413}

or

37.1

St属于logN函数（s*e^ut,s^2*e*(2ut)*(e*(t*sigma^2)-1)）?

1、可以用这个算吗？

2、用这个算完，均值是53.89？

3、用这个算完，方差是58.68？

4、如果上面3个都是对的，那左偏2.58个标准差后是34.16、右偏2.58个标准差后是73.63？

5、我真的搞不太懂ln（St）、St、R这三个分布的关系，就是什么时候题目用哪个？各自的分布中均值和方差我都知道，我也知道其实是可以互换的，可是感觉一道题目如果用不同的分布算出来的数字不一样呀！

添加评论
2 个答案
已采纳答案

袁园_品职助教 · 2021年03月22日

St 是lognormal 不是 normal，所以范围不可以直接用均值加减几倍标准差来算

袁园_品职助教 · 2021年03月21日

同学你好！

因为 stock price 在长时间内是符合 lognormal 分布而不是 normal 分布，所有这里不能直接用 St 的均值和方差来算

海胆君 · 2021年03月21日

我列的那个不就是price属于logN函数吗？

  • 2

    回答

  • 0

    关注

  • 532

    浏览
相关问题

NO.PZ2020021205000061问题如下A stohexpectereturn of 15% ana volatility of 20%. The current priof the stois US50, estimate 99% confinfor the priin six months.Here, the time periois longer, anwe shoulworkwith lognormstributions. From Equations (15.2) an15.3), the logarithm of the stoprihmean:ln(50) + (0.15 - 0.220.2^20.22/2) x 0.5 = 3.9770anstanrviation:0.2*0.5\sqrt{0.5}0.5​ = 0.1414We are 99% certain that:3.9770 - N−1N^{-1}N−1(0.995) x 0.1414 ln(Sr) 3.9770+ N−1N^{-1}N−1 (0.995) X 0.1414or3.6127 ln(Sr) 4.3413so that:e3.6127e^{3.6127}e3.6127 Sr e4.3413e^{4.3413}e4.3413or37.1 Sr 76.8老师好， N−1(0.995) 就是正态分布右尾的分位点2.58对吧？

2024-06-28 15:11 1 · 回答

NO.PZ2020021205000061问题如下A stohexpectereturn of 15% ana volatility of 20%. The current priof the stois US50, estimate 99% confinfor the priin six months.Here, the time periois longer, anwe shoulworkwith lognormstributions. From Equations (15.2) an15.3), the logarithm of the stoprihmean:ln(50) + (0.15 - 0.220.2^20.22/2) x 0.5 = 3.9770anstanrviation:0.2*0.5\sqrt{0.5}0.5​ = 0.1414We are 99% certain that:3.9770 - N−1N^{-1}N−1(0.995) x 0.1414 ln(Sr) 3.9770+ N−1N^{-1}N−1 (0.995) X 0.1414or3.6127 ln(Sr) 4.3413so that:e3.6127e^{3.6127}e3.6127 Sr e4.3413e^{4.3413}e4.3413or37.1 Sr 76.8非标准正态分布关键值是如何计算的。谢谢

2023-03-04 16:22 1 · 回答

NO.PZ2020021205000061 问题如下 A stohexpectereturn of 15% ana volatility of 20%. The current priof the stois US50, estimate 99% confinfor the priin six months. Here, the time periois longer, anwe shoulworkwith lognormstributions. From Equations (15.2) an15.3), the logarithm of the stoprihmean:ln(50) + (0.15 - 0.220.2^20.22/2) x 0.5 = 3.9770anstanrviation:0.2*0.5\sqrt{0.5}0.5​ = 0.1414We are 99% certain that:3.9770 - N−1N^{-1}N−1(0.995) x 0.1414 ln(Sr) 3.9770+ N−1N^{-1}N−1 (0.995) X 0.1414or3.6127 ln(Sr) 4.3413so that:e3.6127e^{3.6127}e3.6127 Sr e4.3413e^{4.3413}e4.3413or37.1 Sr 76.8 N^-1(0.995) 是怎么从99%confince得出来的？能写下过程吗？谢谢。

2022-09-11 09:36 1 · 回答

NO.PZ2020021205000061 从将以来看，sigma*√T，应该是方差吧？我记得quant里学正态分布的时候，不是说这里写的是方差吗？怎么从答案解析来看，是标准差？

2021-03-20 21:25 2 · 回答