关于beta的求法

问题如下：

An investment advisor is analyzing the range of potential expected returns of a new fund designed to replicate the directional moves of the China Shanghai Composite Stock Market Index (SHANGHAI) but with twice the volatility of the index. SHANGHAI has an expected annual return of 7.6% and a volatility of 14.0%, and the risk free rate is 3.0% per year. Assuming the correlation between the fund’s returns and that of the index is 1.0, what is the expected return of the fund using the CAPM?

选项：

A.

12.2%

B.

19.0%

C.

22.1%

D.

24.6%

解释：

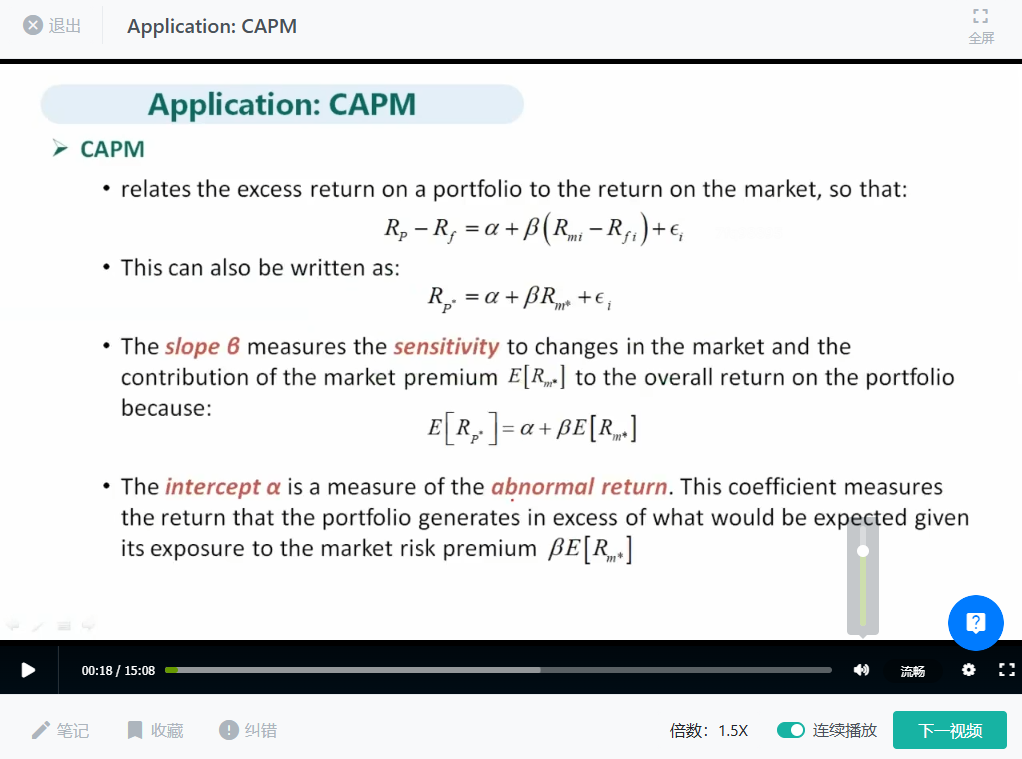
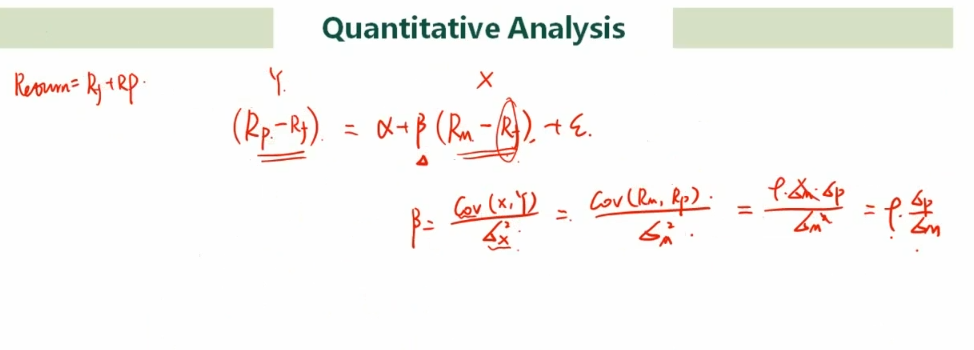
Explanation: If the CAPM holds, then R_i = R_f + β_i * (R_m – R_f).

Beta (β_i ), which determines how much the return of the fund fluctuates in relation to the index return is expressed as follows:

Where i and m denote the new fund and the index, respectively, and R_i = expected return

on the fund, Rm = expected return on the index, R_f = risk-free rate, σ_i = volatility of the fund, σ_m = volatility of the index, Cov(R_i,R_m) = covariance between the fund and the index returns, and Corr(R_i,R_m) = correlation between the fund and the index returns.

If the new fund has twice the volatility of the index, then σ_i = 2σ_i = 2σ_m, and given that Corr(R_i,R_m) = 1.0, the beta of the new fund then becomes:

Therefore, using CAPM, R_i = R_f + β_i * (R_m – R_f) = 0.03 + 2.0*(0.076 – 0.03) = 0.1220 = 12.2%

如果CAPM成立，则Ri = Rf + βi * (Rm - Rf)。
Beta (βi)决定基金收益相对于指数收益波动的程度，表示如下:

其中i和m分别表示新基金和指数，Ri =预期收益
在基金中，Rm =指数的预期收益，Rf =无风险利率，σi =基金的波动率，σm =指数的波动率，Cov(Ri,Rm) =基金与指数收益的协方差，Corr(Ri,Rm) =基金与指数收益的相关性。
如果新基金的波动率是指数的两倍，则σi = 2σi = 2σm，令Corr(Ri,Rm) = 1.0，则新基金的贝塔系数为:

因此，利用CAPM, Ri = Rf + βi *(Rm - Rf) = 0.03 + 2.0*(0.076 - 0.03) = 0.1220 = 12.2%