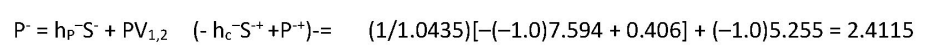这里求p-用的是7.594和0.406

问题如下：

Suppose you observe a non- dividend- paying Australian equity trading for A$7.35. The call and put options have two years to mature, the periodically compounded risk- free interest rate is 4.35%, and the exercise price is A$8.0. Based on an analysis of this equity, the estimates for the up and down moves are u = 1.445 and d = 0.715, respectively.

Calculate the European- style call and put option hedge ratios at Time Step 0 and Time Step 1. Based on these hedge ratios, interpret the component terms of the binomial option valuation model.

选项：

解释：

The computation of the hedge ratios at Time Step 1 and Time Step 0 will require the option values at Time Step 1 and Time Step 2. The terminal values of the options are given in Solution 1.

S⁺⁺ = u²S = 1.445²(7.35) = 15.347

S^+– = udS = 1.445(0.715)7.35 = 7.594

S^{– –} = d²S = 0.715²(7.35) = 3.758

S⁺ = uS = 1.445(7.35) = 10.621

S^– = dS = 0.715(7.35) = 5.255

Therefore, the hedge ratios at Time 1 are

In the last hedge ratio calculation, both put options are in the money (p–+ and p– –). In this case, the hedge ratio will be –1, subject to a rounding error. We now turn to interpreting the model’s component terms. Based on the no- arbitrage approach, we have for the call price, assuming an up move has occurred, at Time Step 1

• C⁺ = h_c⁺S⁺ + PV_1,2 (- h_c⁺S^+- +C^+-)-= 0.9476(10.621) + (1/1.0435)[–0.9476(7.594) + 0.0] = 3.1684

Thus, the call option can be interpreted as a leveraged position in the stock. Specifically, long 0.9476 shares for a cost of 10.0645 [= 0.9476(10.621)] partially financed with a 6.8961 {= (1/1.0435)[–0.9476(7.594) + 0.0]} loan. Note that the loan amount can be found simply as the cost of the position in shares less the option value [6.8961 = 0.9476(10.621) – 3.1684]. Similarly, we have

• C^-= h_c^–S^- + PV_1,2 (- h _c^–S^-- +C^--)-= 0.0(5.255) + (1/1.0435)[–0.0(3.758) + 0.0] = 0.0

Specifically, long 0.0 shares for a cost of 0.0 [= 0.0(5.255)] with no financing.

For put options, the interpretation is different. Specifically, we have

P⁺ =+ h_P⁺S⁺⁺ + PV_1,2 (- h_p⁺S⁺⁺ +p⁺⁺)-= (1/1.0435)[–(–0.05237)15.347 + 0.0] + (–0.05237)10.621 = 0.2140

Thus, the put option can be interpreted as lending that is partially financed with a short position in shares. Specifically, short 0.05237 shares for a cost of 0.55622 [= (–0.05237)10.621] with financing of 0.77022 {= (1/1.0435)[–(–0.05237)15.347 + 0.0]}. Note that the lending amount can be found simply as the proceeds from the short sale of shares plus the option value [0.77022 = (0.05237)10.621 + 0.2140]. Again, we have

P^- = h_P^–S^- + PV_1,2 (- h_c^–S^-+ +P^-+)-= (1/1.0435)[–(–1.0)7.594 + 0.406] + (–1.0)5.255 = 2.4115

Here, we short 1.0 shares for a cost of 5.255 [= (–1.0)5.255] with financing of 7.6665 {= (1/1.0435)[–(–1.0)7.594 + 0.406]}. Again, the lending amount can be found simply as the proceeds from the short sale of shares plus the option value [7.6665 = (1.0)5.255 + 2.4115].

Finally, The interpretations remain the same at Time Step 0: c = h_cS + PV0,1(–hcS^– + c^–) =

The interpretations remain the same at Time Step 0:

C=h_cS+ PV_0,1 (-h_cS^-+C^-)-= 0.5905(7.35) + (1/1.0435)[–0.5905(5.255) + 0.0] = 1.37

Here, we are long 0.5905 shares for a cost of

4.3402 [=0.5905(7.35)] partially financed with a 2.97 {= (1/1.0435)[–0.5905(5.255) + 0.0] or = 0.5905(7.35) – 1.37} loan.

P = h_PS+ PV_0,1 (-h_PS⁺+P⁺)= (1/1.0435){–[–0.4095(10.621)] + 0.214} + (–0.4095)7.35 = 1.36

Here, we short 0.4095 shares for a cost of 3.01 [= (–0.4095)7.35] with financing of 4.37 (= (1/1.0435){–[–0.4095(10.621)] + 0.214} or = (0.4095)7.35 + 1.36).