考的是哪个知识点？

问题如下：

Remington and Montgomery’s first meeting of the day is with a new client, Spencer Shipman, who recently won $900,000 in the lottery. Shipman wants to fund a comfortable retirement. Earning a return on his investment portfolio that outpaces inflation over the long term is critical to him. He plans to withdraw $54,000 from the lottery winnings investment portfolio in one year to help fund the purchase of a vacation home and states that it is important that he be able to withdraw the $54,000 without reducing the initial $900,000 principal. Montgomery suggests they use a risk-adjusted expected return approach in selecting one of the portfolios provided in Exhibit 1.

Exhibit 1 Investment Portfolio One-Year Projections

Which of the portfolios provided in Exhibit 1 has the highest probability of enabling Shipman to meet his goal for the vacation home?

选项：

Portfolio 1

Portfolio 2

Portfolio 3

解释：

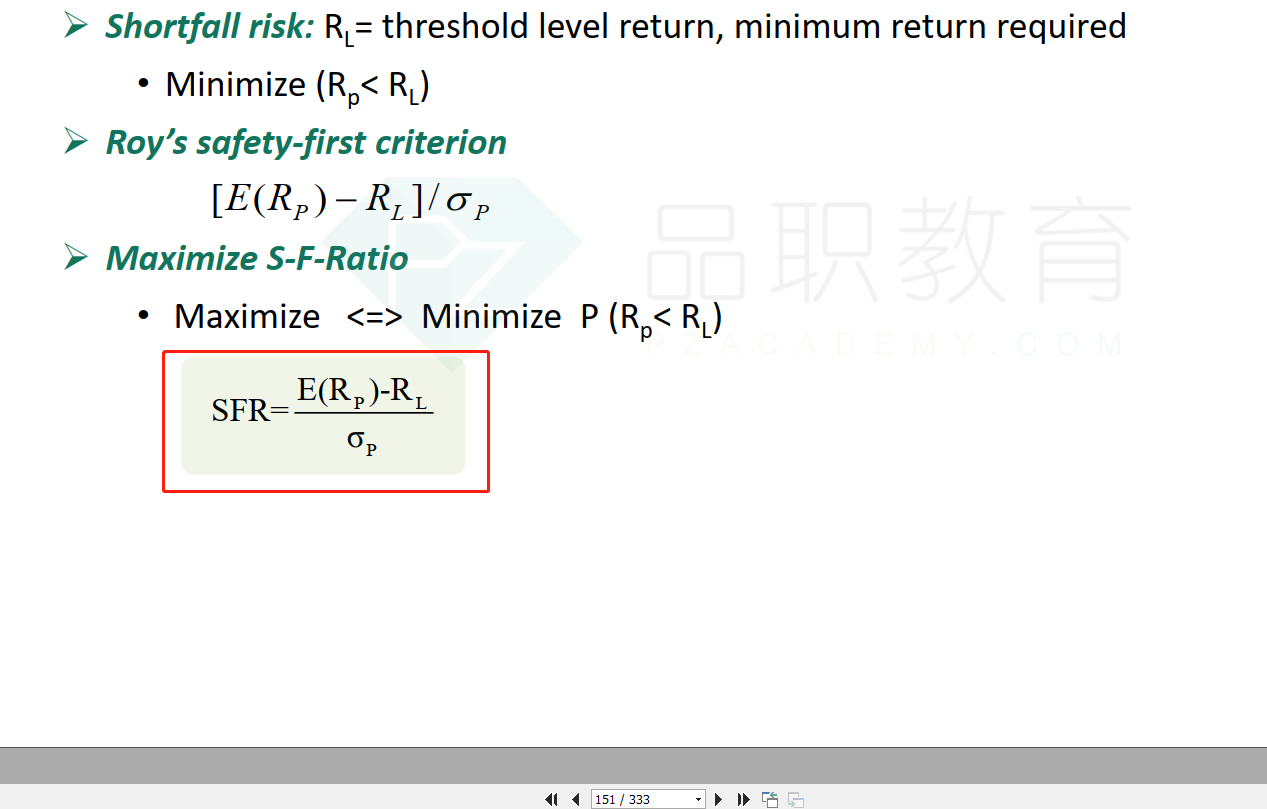
Portfolio 2 has the highest probability of enabling Shipman to meet his goal for the vacation home. All three of the portfolios’ expected returns over the next year exceed the 6.0% (see calculations below) required return threshold to avoid reducing the portfolio. However, on a risk-adjusted basis, Portfolio 2 (probability ratio of 0.231) has a higher probability of meeting and surpassing the threshold than either Portfolio 1 (probability ratio of 0.175) or Portfolio 3 (probability ratio of 0.225).

Step 1: Calculate the required return threshold: 54,000 ÷ 900,000 = 0.06 = 6.0%.

Step 2: To decide which allocation is best for Shipman, calculate the probability ratio:

[E(R_p ) – RL ] ÷ σ_p

Portfolio 1: (10.50% – 6.0%) ÷ 20.0% = 4.50% ÷ 20.0% = 0.225.

Portfolio 2: (9.00% – 6.0%) ÷ 13.0% = 3.00% ÷ 13.0% = 0.231. (Highest)

Portfolio 3: (7.75% – 6.0%) ÷ 10.0% = 1.75% ÷ 10.0% = 0.175.