NO.PZ2023040601000103
问题如下:
Which of the following pairs of weights would be used to achieve the highest Sharpe ratio and optimal amount of active risk through combining the Indigo Fund and benchmark portfolio, respectively?
选项:
A.1.014 on Indigo and –0.014 on the benchmark
1.450 on Indigo and –0.450 on the benchmark
1.500 on Indigo and –0.500 on the benchmark
解释:
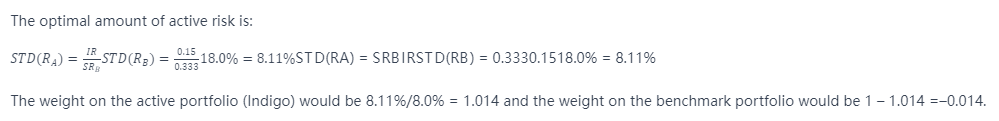
The optimal amount of active risk is:
The weight on the active portfolio (Indigo) would be 8.11%/8.0% = 1.014 and the weight on the benchmark portfolio would be 1 – 1.014 =–0.014.
We can demonstrate that these weights achieve the maximum Sharpe ratio (of 0.365). Note that 8.11% is the optimal level of active risk, and that Indigo has an expected active return of 1.014(1.2%) = 1.217% over the benchmark (and a total excess return of 6.0% + 1.217% = 7.217%. The portfolio total risk is
Taking the square root, STD(RP)= 19.743, and the optimal Sharpe ratio is indeed 7.217/19.743 = 0.365.
此题中解答中total excess return of 6.0% + 1.217% = 7.217%,的6%是如何得到的?