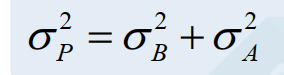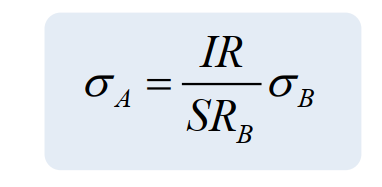active risk-有问必答-品职教育专注CFA ESG FRM CPA 考研等财经培训课程

active risk

NO.PZ2015121810000013

问题如下：

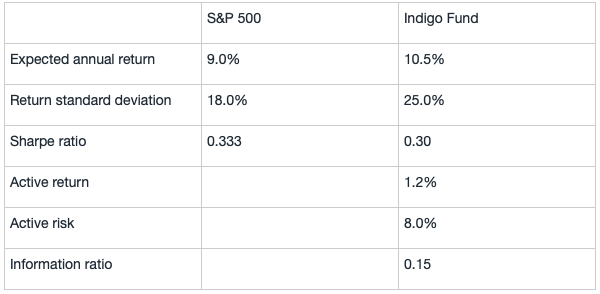
Which of the following pairs of weights would be used to achieve the highest Sharpe ratio and optimal amount of active risk through combining the Indigo Fund and benchmark portfolio, respectively?

选项：

1.014 on Indigo and –0.014 on the benchmark

1.450 on Indigo and –0.450 on the benchmark

1.500 on Indigo and –0.500 on the benchmark

解释：

A is correct.

The optimal amount of active risk is:

\sigma_A=\frac{IR}{SR_B}\sigma_B=\frac{0.15}{0.333}\times18\%=8.11\%

The weight on the active portfolio (Indigo) would be 8.11%/8.0% = 1.014 and the weight on the benchmark portfolio would be 1 – 1.014 = – 0.014.

考点：Optimal amount of active risk

解析：Optimal amount of active risk

$\sigma_A=\frac{IR}{SR_B}\sigma_B=\frac{0.15}{0.333}\times18\%=8.11\%$

Indigo Fund现在的active risk是8%，为了使active risk达到最优水平，就将Indigo Fund与benchmark再做组合，形成active risk最优的combined fund。

假设Indigo Fund的权重为c, 那么

$\sigma_A=c\sigma_A^{fund},\;8.11\%=c8\%,\;c=1.014$

因此，benchmark的权重为1-1.014=-0.014

这道题的思路是根据 sigema （portfolio+benchmark）=C*sigema portfolio这个式子算出C，然后再看是long short。

有几个问题：

1、不太明白optimal amount of active risk算出来的是sigema （portfolio+benchmark），还是sigema portfolio ？

2、请问讲义244页的optimal amount of active risk=12%，是sigema （portfolio+benchmark），还是sigema portfolio？是怎么看出来的？

3、为什么算sigema （portfolio+benchmark）用的是表格中return standard deviation，而算sigema portfolio用的却不是return standard deviation，而是active risk

4、可以用讲义243页关于“sigema P 平方”的那个公式来算sigema portfolio吗？

有点晕，求老师耐心解答，谢谢！

同学你好，

optimal amount of active risk的背景为：①有一个基金经理主动投资的portfolio（即本题的indigo fund），由于是主动投资，所以这个portfolio除了有自己的return standard deviation（下图25.0%）之外，还有一个自己的active risk（下图的8.0%）；②有一个benchmark portfolio（即本题的S&P 500），benchmark没有active risk，但有自己的standard deviation（下图的18.0%）；

optimal amount of active risk是将这两个portfolio重新再组合成一个combined portfolio，达到active risk的最优水平。

combined portfolio除了active risk之外，也有自己的standard deviation，即下面公式计算的σp，但这个考察的很少。

所以，

1）optimal amount of active risk算出来的是combined portfolio的active risk，即sigema （portfolio+benchmark）。而sigema portfolio实际有两个，即本题的25.0%和8.0%，一般指的是后者，也就是主动投资的portfolio的active risk（下面sigma portfolio都代指active risk）。一般题目会直接给出；

2）提到了optimal amount of active risk，对应的就是portfolio+benchmark的combined portfolio。sigema portfolio一般指主动投资的portfolio自己的active risk，不是optimal的。

3）算sigema （portfolio+benchmark）根据的是以下公式，逐项代入即可。sigma portfolio（本题中的8.0%）是因主动管理而带来的active risk。

4）σp的平方是combined portfolio的total risk（standard deviation，不是active risk）。可以算，但是很少有要求计算这部分的。

总之，这个考点常规考法就是本题，掌握了这道题基本上就够用了。

active risk

1 个答案

1

3

710

相关问题