lynn666 · 2023年02月06日

题目中怎么看出来求得是当前的价格？

NO.PZ2018122701000073

问题如下：

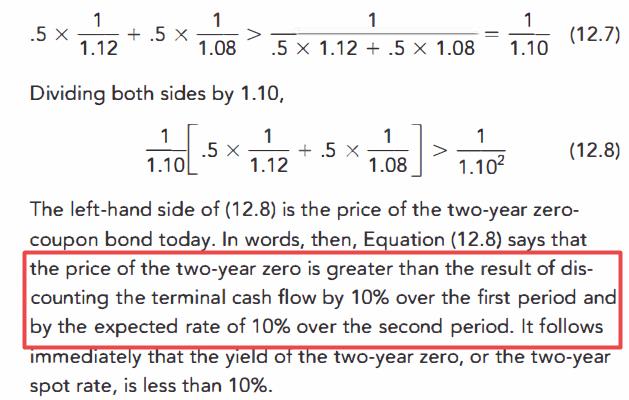
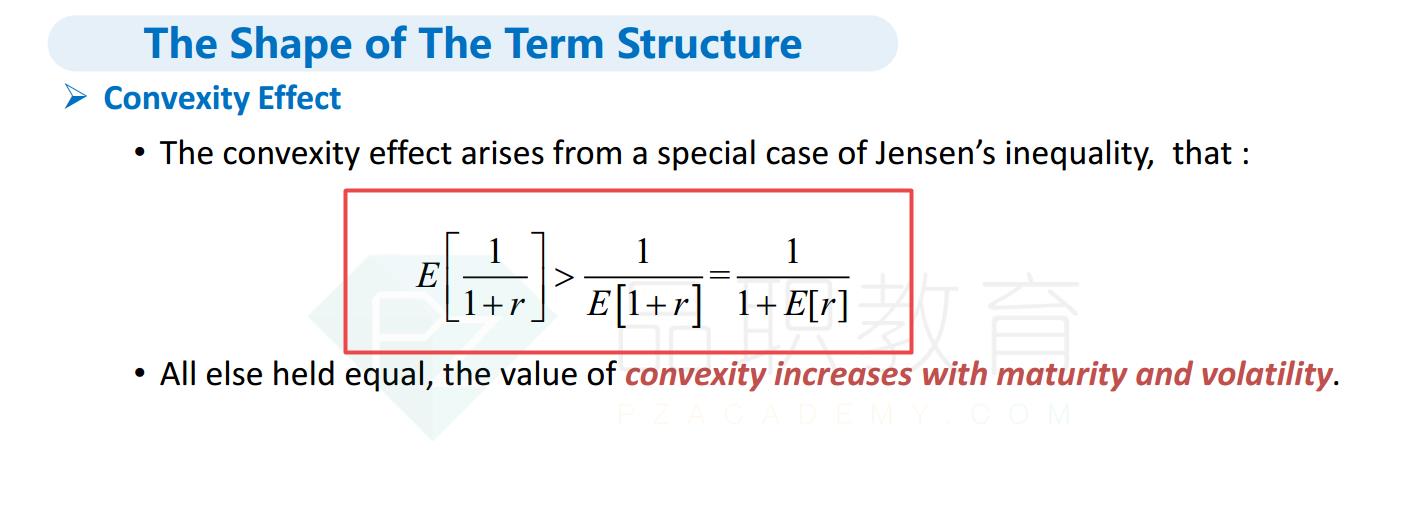
For a 2-year zero-coupon bond, the 1-year rate is expected to remain at 5% for the first year. For the second year, it is foretasted the that 1-year spot rate will be either 7% or 3% at equal probability of 50%. If you are asked to reflect the convexity effect for this 2-year bond by Jensen’s inequality formula, which of the following inequalities is the best answer?

选项：

$0.90736 > $0.90703.

$0.90703 > $0.90000.

$0.95238 > $0.90736.

$0.95273 > $0.95238

解释：

A is correct.

考点：Jensen's inequality formula

解析：

不等式左边

$E(\frac1{1+r})=0.5\times\frac1{1.07}+0.5\times\frac1{1.03}=0.95273$

0.95273/1.05 = 0.90736

不等式右边

$\frac{\$1}{0.5\times1.07+0.5\times1.03}=\frac{\$1}{1.05}=0.95238$

0.95238/1.05 = 0.90703

如题，怎么确定要不要再除以1.05%折现回0时刻呢？

添加评论

2 个答案

李坏_品职助教 · 2023年02月06日

嗨，爱思考的PZer你好：

这个题是从原版书改过来的，原版书的说法如下：

这里用的也是折现到0时刻的现值，这样才是零息债券的价格，他用价格来表示inequality的不等式关系。其实不除以1.05也不影响inequality不等式，题目的本意是为了和原版书保持一致。

----------------------------------------------
虽然现在很辛苦，但努力过的感觉真的很好，加油！

添加评论

李坏_品职助教 · 2023年02月06日

嗨，努力学习的PZer你好：

题目里问的是 Jensen’s inequality formula，这个公式：

所以题目问的就是要我们尽量凑出来这个不等式左右两边的结果，来证明这个不等式是成立的。

左边是E[1/1+r]，题目里说r要么是3%，要么是7%，所以是对(1/1+r) 这个整体求均值，概率各自都是50%。所以是50% * (1/1.07) + 50% * (1/1.03)。

右边是1/ E[1+r]，等于是1/(1+r)的均值，分子是1，分母是1+r的均值。由于r要么是3%要么是7%，所以1+r的均值也就是50% * 1.03 + 50% *1.07，所以右边就是1/ (50% * 1.03 + 50% *1.07)

这就是完全按照jensen inequality的不等式来算的。

----------------------------------------------
虽然现在很辛苦，但努力过的感觉真的很好，加油！

添加评论

lynn666 · 2023年02月06日

我通过jensen不等式算出了题目解析里的0.95273和0.95238这两个数。但是做题的时候就不确定要不要再除以1.05，怎么确定要不要再除以 1.05呢？

题目中怎么看出来求得是当前的价格？

2 个答案

2

0

292

相关问题