开发者:上海品职教育科技有限公司 隐私政策详情

应用版本:4.2.11(IOS)｜3.2.5(安卓)APP下载

返回

上小学 · 2023年02月05日

在多元回归方程总，R SQUARE 是否还等于CORRELATION 的平方？

  1. FRM I
  2. Quantitative

NO.PZ2020010801000036

问题如下：

In a model with two explanatory variables, Yi=α+β1X1i+β2X2i+ϵiY_i = \alpha + \beta_1X_{1i} +\beta_2X_{2i} + \epsilon_i, what does the correlation need to be between the two regressors, X1 and X2, for the variance inflation factor to be above 10?

选项：

解释：

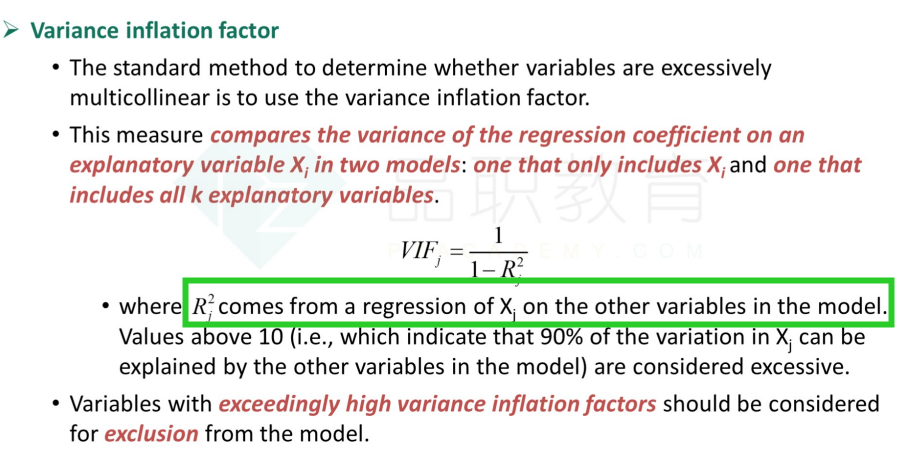
The variance inflation factor is 11−Rj2\frac{1}{1-R_j^2}, where Rj2R_j^2 measures how well variable j is explained by the other variables in the model. Here there are two variables, and so Rj2=ρX1X22R_j^2=\rho_{X_1X_2}^2. The variance inflation factor of 10 solves 10=1/(1−ρX1X22)10 = 1/(1 - \rho_{X_1X_2}^2 ), so that 1/(1−ρX1X22)=1/101/(1 - \rho_{X_1X_2}^2 ) = 1/10 or ρX1X22=1−1/10=0.9\rho_{X_1X_2}^2=1-1/10=0.9.

Correlations greater than (in absolute value) 0.9≈0.948\sqrt 0.9 ≈ 0.948 would produce values above 10.

在课程中讲到，在多元回归方程中，R SQUARE等于CORRELATION 的平方是不成立的，这是多元和一元回归的一个区别。因此，在本题目中，对于CORRELATION 没有任何思路，只计算出一个0.9,然后再也无法继续下去了。还麻烦请详细讲讲 R SQUARE 到底是否等于 CORRELATION 的平方，非常感谢！

添加评论
1 个答案

pzqa27 · 2023年02月05日

嗨，从没放弃的小努力你好：


同学您好，这里的R^2和您想象的R^2不是一个东西，不是一个考点。

您所想象的R^2是goodness of fit，是X能够解释Y的程度

这个题里的R^2是解释变量Xj与其他解释变量X的关系，这个点在讲义231页，section6 serial correlation这个视频2倍速7min有讲解，同学可以再回去听一下。

----------------------------------------------
努力的时光都是限量版，加油！

  • 1

    回答

  • 0

    关注

  • 300

    浏览
相关问题

NO.PZ2020010801000036 问题如下 In a mol with two explanatory variables, Yi=α+β1X1i+β2X2i+ϵiY_i = \alpha + \beta_1X_{1i} +\beta_2X_{2i} + \epsilon_iYi​=α+β1​X1i​+β2​X2i​+ϵi​, whes the correlation neeto between the two regressors, X1 anX2, for the varianinflation factor to above 10? The varianinflation factor is 11−Rj2\frac{1}{1-R_j^2}1−Rj2​1​, where Rj2R_j^2Rj2​ measures how well variable j is explainethe other variables in the mol. Here there are two variables, anso Rj2=ρX1X22R_j^2=\rho_{X_1X_2}^2Rj2​=ρX1​X2​2​. The varianinflation factor of 10 solves 10=1/(1−ρX1X22)10 = 1/(1 - \rho_{X_1X_2}^2 )10=1/(1−ρX1​X2​2​), so th1/(1−ρX1X22)=1/101/(1 - \rho_{X_1X_2}^2 ) = 1/101/(1−ρX1​X2​2​)=1/10 or ρX1X22=1−1/10=0.9\rho_{X_1X_2}^2=1-1/10=0.9ρX1​X2​2​=1−1/10=0.9. Correlations greater th(in absolute value) 0.9≈0.948\sqrt 0.9 ≈ 0.9480​.9≈0.948 woulprovalues above 10. 这个知识点在新考纲范围内吗

2024-05-11 15:31 1 · 回答

NO.PZ2020010801000036

2022-01-10 01:02 1 · 回答

NO.PZ2020010801000036 考察的是哪个知识点呢？谢谢！

2021-09-21 08:28 2 · 回答

 varianinflation factor这个知识点在基础班哪里提到的呀？一点印象也没有了。谢谢老师

2020-08-29 18:04 1 · 回答