NO.PZ2022071202000037
问题如下:
Question
An analyst has established the following prior probabilities regarding a company's next quarter's earnings per share (EPS) exceeding, equaling, or being below the consensus estimate.

Several days before releasing its earnings statement, the company announces a cut in its dividend. Given this new information, the analyst revises his opinion regarding the likelihood that the company will have EPS below the consensus estimate. He estimates the likelihood the company will cut the dividend, given that EPS exceeds/meets/falls below consensus, as reported below.

The analyst thus determines that the unconditional probability for a cut in the dividend, P(Cut div), is equal to 23.75%. Using Bayes’ formula, the updated (posterior) probability that the company’s EPS are below the consensus is closest to:
选项:
A.85%. B.72%. C.20%.解释:
Solution
B is correct. Bayes’ Formula:Updated probability of event given the new information=Probability of the new information given event Unconditional probability of the new information×Prior probability of eventwhere
Updated probability of event given the new information: P(EPS below|Cut div);
Probability of the new information given event: P(Cut div|EPS below) = 85%;
Unconditional probably of the new information: P(Cut div) = 23.75%;
Prior probability of event: P(EPS below) = 20%.
Therefore, the probability of EPS falling below the consensus is updated as:
P(EPS below|Cut div) = [P(Cut div|EPS below)/P(Cut div)] × P(EPS below)
= (0.85/0.2375) × 0.20 = 0.71579 ~ 72%
B is incorrect. It is the given P(Cut div|EPS below).
C is incorrect. It simply multiplies the unconditional probability for a cut in the dividend with the conditional probability of a cut in the dividend given that EPS falls below consensus: P(Cut div) × P(Cut div|EPS below) = 0.2375 × 0.85 = 20.188.%
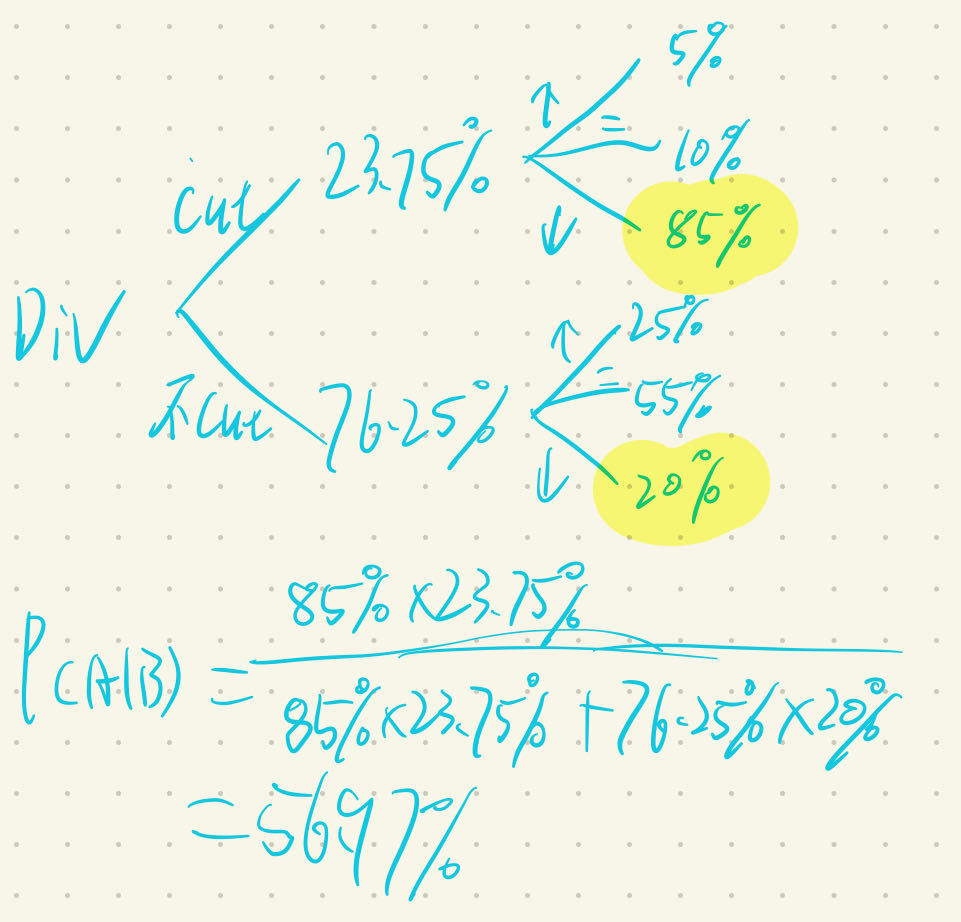
用老师上课讲的画图法做的,请问哪里出了问题



