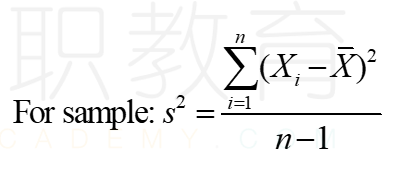第一个问题。为啥必须用算术平均而不是几何平均，我的思路是先算五年总收益开五次跟号转换年化，请老师指正一下我的错误根本原因是哪个点-有问必答-品职教育专注CFA ESG FRM CPA 考研等财经培训课程

第一个问题。为啥必须用算术平均而不是几何平均，我的思路是先算五年总收益开五次跟号转换年化，请老师指正一下我的错误根本原因是哪个点

NO.PZ2015121802000021

问题如下：

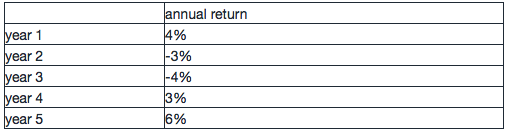
Calculate the standard deviation of annual returns on an investment based on the following chart:

选项：

1.2%.

4.44%.

19.7%.

解释：

B is correct.

Mean annual return = (4% - 3% - 4% + 3% + 6%) / 5 = 1.2%

Squared deviations from the mean:

4%-1.2%=2.8% （2.8%）²= 7.84

-3% -1.2% = -4.2% （-4.2%）² = 17.64

-4% -1.2% = -5.2% （-5.2%）² = 27.04

3% -1.2% =1.8% （1.8^%）²= 3.24

6% -1.2% = 4.8% （4.8%） ²= 23.04

Sum of squared deviations = 7.84 + 17.64 + 27.04 + 3.24+ 23.04 = 78.8

Sample variance = 78.8/(5 - 1) = 19.7

Sample standard deviation = 19.7^1/2= 4.44%

第二个问题，为啥sample是除以n-1，数量学科有这个公式但太久了记不清了，谢谢老师

嗨，从没放弃的小努力你好：

1.同学你好，这道题求的是标准差。计算标准差时候的mean，都用算术平均。这是约定俗成的计算方法。

2.这个问题最好还是记住。

样本方差计算公式里分母为n-1的目的是为了让方差的估计是无偏的。

原因解释

1、设若总体数据已知，则该总体的数字特征不存在推测的问题，只存在描述的问题，是故总体方差计算公式中的除数应为“N”。

2、以“n-1”为除数的样本方差计算公式是总体方差的无偏估计值计算式。

3、以“n”为除数的样本方差计算公式是总体方差的渐近无偏估计值计算式。

4、如果只是要描述样本数据间的离散程度，则样本方差计算公式中的除数应为“n”。

5、当n足够大的时候，不必太在意样本方差计算公式中除数的这两种不同的选择。

6、在多数场合，习惯上总是采用以“n-1”为除数的样本方差计算方式。

----------------------------------------------
努力的时光都是限量版，加油！

第一个问题。为啥必须用算术平均而不是几何平均，我的思路是先算五年总收益开五次跟号转换年化，请老师指正一下我的错误根本原因是哪个点