NO.PZ2020011303000225
问题如下:
Suppose that the five-, ten-, and 30-year rates are 4%, 5%, and 6% with semiannual compounding. Calculate the duration and convexity of zero-coupon bonds with five-, ten-, and 30-years to maturity. What position in five- and 30-year bonds would have a duration equal to that of the ten-year bond? Compare the convexities of (a) the positions in the ten-year bond and (b) the position in the five- and 30-year bonds? Which of these positions will give the better return if (a) rates remain the same and (b) there are parallel shifts in the term structure?
选项:
解释:
The duration and convexities calculated by making one-basis-point changes are 
We can construct a bond with a duration of 9.756 by investing β in the five-year
bond and 1−β in the 30-year bond where:
4.902β+29.126(1-β)=9.756
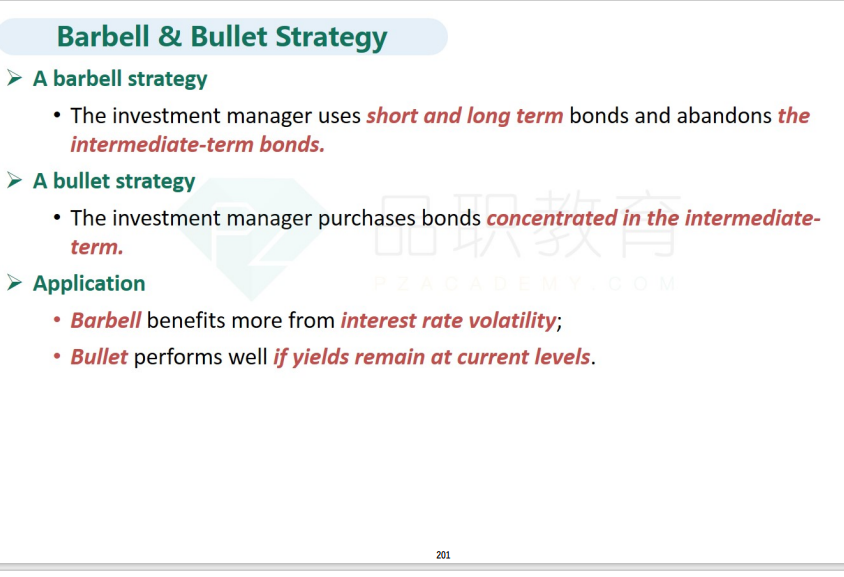
β is 0.7996, which we round to 0.8. We therefore invest 80% in the five-year bond and 20% in the 30-year bond. The ten-year bond investment (a bullet) has a convexity of 99.941 whereas the portfolio of five- and 30-year bonds (a barbell) has a convexity of about:
0.8×26.423+ 0.2×862.472 = 193.6
If rates remain the same the bullet will provide a yield of 5%, whereas the
barbell will provide a weighted average yield of 0.8 × 4 + 0.2 × 6 or 4.4%. The bullet will perform
better. When there are parallel shifts to the term structure, this effect is mitigated
somewhat by the barbell’s higher convexity, which leads to an immediate
improvement in the value of the barbell position. However, the bullet will
perform better for some non-parallel shifts.
The bullet will perform better. When there are parallel shifts to the term structure, this effect is mitigated somewhat by the barbell’s higher convexity, which leads to an immediate improvement in the value of the barbell position. However, the bullet will perform better for some non-parallel shifts.