NO.PZ2019011002000009
问题如下:
Bond C is a 4-year corporate bond. The bond is a floating rate bond and its coupon rate is the one-year benchmark rate plus 4%. Assume the risk-neutral probability of default (the hazard rate) for each date for the bond is 1.50%, and the recovery rate is 25%.
The current spot rates and forward rates are shown in the table below:

Li, a credit analyst in a wealth management firm, believes that the future interest rate volatility is 20%.
He constructed a binomial interest rate tree by using his volatility estimation and the current yield curve.
The binomial interest rate tree is shown below:

The market price of this floating rate bond is 1054 currently. According to the information above, compared with the bond’s fair value, the value of the bond is:
选项:
A.
Undervalued
B.
Overvalued
C.
Fairly-valued
解释:
A is correct.
考点:使用二叉树对有风险的浮动利率债券进行估值
解析:
本题是要计算Floating-rate bond的Fair value;首先需要用二叉树模型计算其VND,有:

该浮动利率债券的Coupon为Benchmark rate加上4%,因此Date 4的Coupon rate出现的情况有:
8.0804%+4%;5.4164%+4%;3.6307%+4%;2.4338%+4%
因此Date 4现金流的情况:
1000×(1+0.080804+0.04)=1120.80
1000×(1+0.054164+0.04)=1094.16
1000×(1+0.036307+0.04)=1076.31
1000×(1+0.024338+0.04)=1064.34
由Date 4的现金流和二叉树所示利率,可以折现求得Date 3四个节点的Value:
1120.80/1.080804=1037.01
1094.16/1.054164=1037.94
1076.31/1.036307=1038.60
1064.34/1.024338=1039.05
由Date 2的Benchmark利率可以知道在Date 3三个节点Coupon rate出现的情况有:
4.3999%+4%;2.9493%+4%;1.9770%+4%
因此Date 3 Coupon现金流的情况:
1000×(0.043999+0.04)=84
1000×(0.029493+0.04)=69.49
1000×(0.019770+0.04)=59.77
将Date 3各个节点的Coupon加上Date 3各个节点的Value构成Date 3的总现金流,利用二叉树向Date 2折现:
[(0.5×1037.01+0.5×1037.94)+84]/1.043999=1074.21
[(0.5×1037.94+0.5×1038.60)+69.49]/1.029493=1076.03
[(0.5×1038.60+0.5×1039.05)+59.77]/1.019770=1077.30
Date 2的两个节点的Coupon由Date 1 Benchmark利率决定,因此Date 1的Coupon rate出现的情况有:
2.1180%+4%;1.4197%+4%;
因此Date 2 coupon现金流的情况:
1000×(0.021180+0.04)= 61.18
1000×(0.014197+0.04)= 54.20
Date 2的Coupon现金流加上Value现金流构成Date 2的总现金流向Date 1折现:
(1074.21×0.5+1076.03×0.5+61.18)/(1+2.1180%)=1112.73
(1076.03×0.5+1077.30×0.5+54.20)/(1+1.4197%)=1115.03
Date 1的Coupon由Date 0时刻Benchmark利率决定,因此Date 1的Coupon rate有:
-0.25%+4%
则Date 1的Coupon为:
1000×(-0.0025+0.04)= 37.50
Date 1的Coupon现金流加上Value现金流构成Date 1的总现金流向Date0折现:
(1112.73×0.5+1115.03×0.5+37.50)/(1-0.25%)=1154.27
这个1154.27为债券的VND;下面利用二叉树计算债券的CVA;
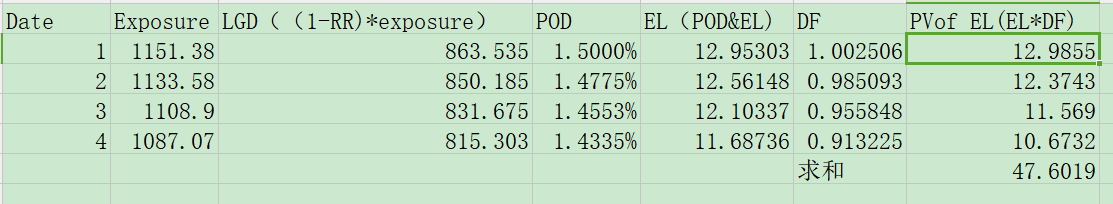
Date 4的Exposure为:
0.125×1120.80+0.375×1094.16+0.375×1076.31
+0.125×1064.34=1087.07
Date 3的Exposure为:
0.125×1037.01+0.375×1037.94+0.375×1038.60
+0.125×1039.05+0.250×84+0.5×69.49+0.250×59.77=1108.90
Date 2的Exposure为:
0.25×1074.21+0.5×1076.03+0.25×1077.30
+61.18×0.5+54.20×0.5=1133.583
Date 1的Exposure为:
1112.73×0.5+1115.03×0.5+37.50=1151.38
由以上Exposure数据,已知Recovery rate为25%,所以可以知道Recovery;再用Exposure减去Recovery可以得到Loss given default (LGD);本题 hazard rate为1.5%,则可以算出POS以及对应POD;再用违约损失LGD乘以违约概率POD得到预期损失Expected loss;Expected loss通过折现因子求得PV(EL);加总即得到债券的CVA;

因此由债券的VND减去其CVA可以的到Fair value:
1154.27 – 47.6019 = 1106.67;已知当前债券的市场价格为1054;则可以改债券是相对被低估的。
请问下本题CVA 中exposure具体求解步骤